Andriy Haydys - G₂ instantons and the Seiberg Witten monopoles

Sung-Soo Kim, 5d Seiberg-Witten curve through toric-like diagramПодробнее

Hiraku Nakajima - Donaldson = Seiberg-Witten from Mochizuki's formula and instanton countingПодробнее

Electroweak-Symmetric Dark Monopoles from Preheating - Nicholas OrlofskyПодробнее

The 3d A-model: generalized Seiberg-Witten equations, vortices and monopoles, J. Hilburn Part 1/3Подробнее

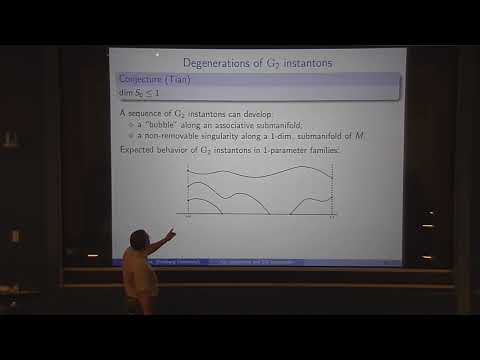
Andriy Haydys - On degenerations of the Seiberg–Witten monopoles and G₂ instantonsПодробнее

Andriy Haydys - Seiberg-Witten monopoles and flat PSL(2,R) connectionsПодробнее

Instantons and Monopoles (Lecture 2) by Sergey CherkisПодробнее

Hiraku Nakajima - Donaldson = Seiberg-Witten from Mochizuki's formula and instanton countingПодробнее

蔡宗軒, On Higgs Branch Localization of Seiberg-Witten Theories on EllipsoidПодробнее

New results on magnetic monopolesПодробнее

Monopoles and Vortices in 3d N=4 gauge theoriesПодробнее

Semi-infinite Hodge structure: from BCOV theory to Seiberg-Witten geometryПодробнее

Semi-infinite Hodge structure: from BCOV theory to Seiberg-Witten geometry - Si LiПодробнее

A hitchin-kobayashi correspondance for generalized seiberg-witten equations by Varun ThakreПодробнее

Introduction to Seiberg-Witten Theory - Dr Elli Pomoni (Lecture 1)Подробнее

Seiberg-Witten Theory for Families 2Подробнее